Welcome Back! November 29th

Algebra I

Today we discussed
We reviewed multiplying polynomials and extended the conversation to include special products:  product of differences, sum of squares and sum of differences.  Then, we moved on to factors and Greatest Common Factor (GCF).

Recall:

Square of a Sum:  (a + b)² = a² + 2ab + b²

Square of a Difference:  (a - b)² = a² - 2ab + b²

Product of Sum & Difference:  (a + b)(a - b) = a² -  b²

Sections Covered in Textbook
1) 6-9: Some Special Products (pages 243-246)
2) 7-1: Factors and Greatest Common Factor (GCF) (pages 256-260)

Video Tutorials
1) Using the formula for finding the product of a sum and difference.
http://www.virtualnerd.com/algebra-1/polynomials-and-factoring/special-products/sum-difference-product/sum-and-difference-product-formula-example
2) Finding the square of a sum.
http://www.virtualnerd.com/algebra-1/polynomials-and-factoring/special-products/squares-of-sum-difference/square-of-a-sum-formula

Assignments
1) Page 245 #'s 11-35 odd, 36.
2) Pages 258-259 #'s 17-66 - problems in 1st column only!

**PLEASE CHECK ODD ANSWER IN BACK OF TEXTBOOK

---

Pre-Algebra

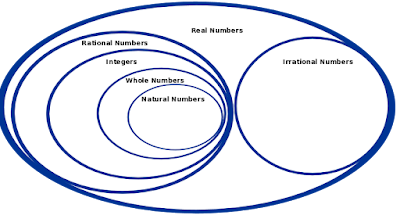
Today we discussed
We briefly reviewed multiplying powers with the same base and then started our conversation about the various sets of numbers (Natural, Whole, Integers, Rational, Real).  From there we discussed how to turn a fraction into a decimal and a decimal into a fraction.

To turn a decimal into a fraction:
1)  Say it
     2)  Write it
          3) Simplify it!

Number Sets:

Sections Covered in Textbook
1) 5-1: Rational Numbers (pages 166-168)
2) 5-2: Writing Decimals as Fractions (pages 169-171)

Video Tutorials
1) Writing Decimals as Fractions
http://www.virtualnerd.com/middle-math/decimals/representing/rewrite-as-fraction-example

Assignments
1) Page 171 #'s 19-43 odd

**PLEASE CHECK ODD ANSWER IN BACK OF TEXTBOOK